Chromatography simulation using mechanistic models facilitates intra-column understanding.
Mechanistic models are based on physical and biochemical effects, written in mathematical language. Many process parameters are directly included in the model equations, and process quality attributes can be calculated from the simulation results. In this way, parameter studies allow the examination of positive or negative effects on the process before they occur in production.
The result of a chromatography simulation is a more than ordinary chromatogram. Mechanistic models capture the movement of molecules along their journey from the interstitial volume of the mobile phase to the pore volume of the mobile phase, and finally the volume of the stationary phase.
Fig 1. Movement of a molecule through a column. From left to right: interstitial volume of the mobile phase, pore volume of the mobile phase, and volume of the stationary phase.
Flow rate induced convection
The molecules injected into the chromatography column are transported with the fluid flow which is induced by a connected pump. The effect of convection is visible by a shifted peak in time. Pumping with a higher velocity leads to higher convection. This can be seen in an earlier peak, where the flowrate defines the position of the peak. Plotted over volume, the peak position and shape might change as well.
An increased velocity will lead to less peak broadening and will also affect mass transfer processes. Apart from the pump speed, a second cause for changes in the flow rate can be varying packing quality. Mechanistic models consider the porosity of the chromatography column as a model parameter which influences the linear flow rate inside the column. The denser the packing, the higher the linear flow.
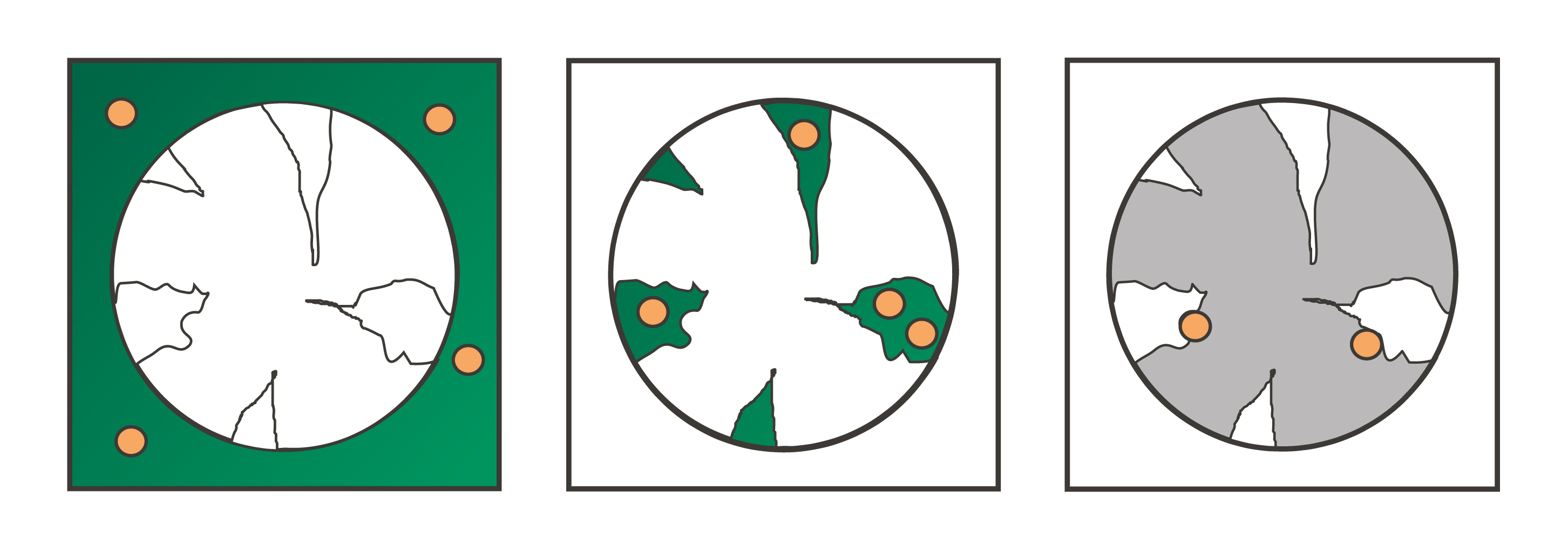
Column packing quality and dispersion
Dispersion consists of different flow effects outside the particle’s pores, such as flow distributors, wall effects, turbulence, eddy diffusion, and molecular diffusion. These random diffusive movements and non-ideal flow patterns cause peak broadening. Compared to the convectional effects, dispersive forces have a greater influence on the shape of the chromatogram.
Dispersion is a time-dependent process. The longer molecules stay inside the column, the longer they are affected by dispersion. When assessing column packing quality with a tracer pulse, a slight tailing is a natural result of the peak front being less affected by dispersion in comparison to the back slope which needs longer to pass through the column.
Flow rate dependent pore accessibility and slow pore diffusion
Given that the pore is accessible, a molecule can pass a particle’s stagnant fluid film and enter the particle’s pore volume. The resulting transition between interstitial volume and pore volume is also denoted as a mass transfer from one volume to the other. This transfer can also be velocity-dependent.
In the case of a high flow rate, the likelihood of a molecule passing a bead and not entering its pore system is higher. Once a molecule has entered a particle’s pore, its movement is dominated mainly by diffusion. Both pore accessibility and slow pore diffusion lead to asymmetric or tailed peaks.
Raw material and upstream variability influencing adsorption
Finally, adsorption onto free binding sites, leads to the final shape of your chromatogram. Large molecules such as antibodies usually bind to several ligands at once. Most chromatography models capture this behavior by considering multi-point binding. The concentration of ligands is described with the help of the resin capacity that is provided by most manufacturers.
Competition for free binding sites plays another integral part of chromatography and is also captured by chromatography models. Competitive adsorption naturally depends on the individual concentrations of all species. A change in upstream conditions leading to a different feed stock composition can be simulated directly without recalibrating the model. The same holds true for a change in binding and elution conditions by varying, for example, salt concentrations and pH in silico.